Regularization

Shan-Hung Wu & DataLab
Fall 2024

Regularization refers to techniques that improve the generalizability of a trained model. In this lab, we will guide you through some common regularization techniques such as weight decay, sparse weight, and validation.

Learning Theory

Learning theory provides a means to understand the generalizability of trained model. As explained in the lecture, it turns out that model complexity plays a crucial role: too simple a model leads to high bias and underfitting because it cannot capture the trends or patterns in the underlying data generating distribution; while too complex a model leads to high variance and overfitting because it captures not only the trends of the underlying data generating distribution but also some patterns local to the training data.

Let's see the problems of overfitting and underfitting from a toy regression problem. Suppose we know the underlying data generating distribution: $$\mathrm{P}(\mathrm{x}, \mathrm{y})=\mathrm{P}(\mathrm{y}\,|\,\mathrm{x})\mathrm{P}(\mathrm{x}),$$where$$\mathrm{P}(\mathrm{x})\sim\mathrm{Uniform}$$and$$\mathrm{y}= \sin(x) + \epsilon, \epsilon\sim\mathcal{N}(0,\sigma^2)$$ We can generate a synthetic dataset as follows:

%matplotlib inline
from pylab import *
from sklearn.model_selection import train_test_split

import os
if not os.path.exists("output/") : os.mkdir("output/")
if not os.path.exists("data/") : os.mkdir("data/")

import warnings
warnings.filterwarnings("ignore")

def gen_data(num_data, sigma):
    x = 2 * np.pi * (np.random.rand(num_data) - 0.5)
    y = np.sin(x) + np.random.normal(0, sigma, num_data)
    return (x, y)

num_data = 30
sigma = 0.2
x, y = gen_data(num_data, sigma)
x_train, x_test, y_train, y_test = train_test_split(
        x, y, test_size=0.3, random_state=0)

plt.scatter(x_train, y_train, color='blue')
plt.scatter(x_test, y_test, color='green')

x_grid = np.linspace(-1*np.pi, 1*np.pi)
sin_x = np.sin(x_grid)
plt.plot(x_grid, sin_x, color ='red', linewidth = 2)

plt.xlabel('x')
plt.ylabel('y')
plt.xlim(-np.pi, np.pi)
plt.ylim(-2, 2)
plt.tight_layout()
plt.savefig('./output/fig-dataset-sin.png', dpi=300)
plt.show()

The blue points are training examples and the green ones are testing points. The red curve is the $\sin$ function, which is the function $f^*$ with minimal generalization error $C[f^*]$ (called the Bayes error).

In regression, the degree $P$ of a polynomial (polynomial regression) and the depth of a decision tree (decision tree regression) are both hyperparameters that relate to model complexity. Let's consider the polynomial regression here and fit polynomials of degrees $1$, $5$, and $10$ to 20 randomly generated training data of the same distribution:

from sklearn.preprocessing import PolynomialFeatures
from sklearn.linear_model import LinearRegression
from sklearn.metrics import r2_score

degree = [1, 3, 10]
std_list = []
for d in degree:
    X_fit = np.arange(-np.pi, np.pi, .1)[:, np.newaxis]
    poly = PolynomialFeatures(degree=d)
    
    for i in range(20):
        x, y = gen_data(num_data, sigma)
        x_train, x_test, y_train, y_test = train_test_split(
            x, y, test_size=0.3, random_state=0)
        
        regr = LinearRegression()
        regr = regr.fit(poly.fit_transform(x_train[:,np.newaxis]), 
                        y_train[:,np.newaxis])

        y_fit = regr.predict(poly.transform(X_fit))
        plt.plot(X_fit, y_fit, 
                 color='green', lw=1)
    
    x_grid = np.linspace(-1*np.pi, 1*np.pi)
    sin_x = np.sin(x_grid)
    plt.plot(x_grid, sin_x, color='red', linewidth = 2)
    
    plt.title('Degree: %d' %d)
    plt.xlim(-np.pi, np.pi)
    plt.ylim(-2, 2)
    plt.tight_layout()
    plt.savefig('./output/fig-polyreg-%d.png' % d, dpi=300)
    plt.show()

When $P=1$, the polynomial is too simple to capture the trend of the $\sin$ function. On the other hand, when $P=10$, the polynomial becomes too complex such that it captures the undesirable patterns of noises.

NOTE: regression is bad at extrapolation. You can see that the shape of a fitted polynomial differs a lot from the $\sin$ function outside the region where training points reside.

Error Curves and Model Complexity

One important conclusion we get from learning theory is that the model complexity plays a key role in generalization performance of a model. Let's plot the training and testing errors over different model complexities in our regression problem:

from sklearn.metrics import mean_squared_error

num_data = 50
x, y = gen_data(num_data, sigma)
x_train, x_test, y_train, y_test = train_test_split(
        x, y, test_size=0.3, random_state=0)

mse_train = []
mse_test = []

max_degree = 12

for d in range(1, max_degree):
    poly = PolynomialFeatures(degree=d)
    X_train_poly = poly.fit_transform(x_train[:,newaxis])
    X_test_poly = poly.transform(x_test[:,newaxis])
    
    regr = LinearRegression()
    regr = regr.fit(X_train_poly, y_train)
    y_train_pred = regr.predict(X_train_poly)
    y_test_pred = regr.predict(X_test_poly)
    
    mse_train.append(mean_squared_error(y_train, y_train_pred))
    mse_test.append(mean_squared_error(y_test, y_test_pred))
    

plt.plot(range(1, max_degree), mse_train, label = 'Training error', color = 'blue', linewidth = 2)
plt.plot(range(1, max_degree), mse_test, label = 'Testing error', color = 'red', linewidth = 2)
plt.legend(loc='upper right')
plt.xlabel('Model complexity (polynomial degree)')
plt.ylabel('$MSE$')
plt.tight_layout()
plt.savefig('./output/fig-error-curve.png', dpi=300)
plt.show()

We can see that the training error (blue curve) decrease as the model complexity increases. However, the testing error (red curve) decreases at the beginning but increases latter. We see a clear bias-variance tradeoff as discussed in the lecture.

Learning Curves and Sample Complexity

Although the error curve above visualizes the impact of model complexity, the bias-variance tradeoff holds only when you have sufficient training examples. The bounding methods of learning theory tell us that a model is likely to overfit regardless of it complexity when the size of training set is small. The learning curves are a useful tool for understanding how much training examples are sufficient:

def mse(model, X, y):
    return ((model.predict(X) - y)**2).mean()

from sklearn.model_selection import learning_curve

num_data = 100
sigma = 1
degree = [1, 3, 10]
x, y = gen_data(num_data, sigma)
for d in degree:
    poly = PolynomialFeatures(degree=d)
    X = poly.fit_transform(x[:,np.newaxis])

    lr = LinearRegression()
    train_sizes, train_scores, test_scores = learning_curve(estimator=lr, X=X, y=y, scoring=mse)

    train_mean = np.mean(train_scores, axis=1)
    train_std = np.std(train_scores, axis=1)
    test_mean = np.mean(test_scores, axis=1)
    test_std = np.std(test_scores, axis=1)

    plt.plot(train_sizes, train_mean,
        color='blue', marker='o',
        markersize=5,
        label='Training error')
    plt.fill_between(train_sizes,
        train_mean+train_std,
        train_mean-train_std,
        alpha=0.15, color='blue')
    
    plt.plot(train_sizes, test_mean,
        color='green', linestyle='--',
        marker='s', markersize=5,
        label='Testing error')
    plt.fill_between(train_sizes,
        test_mean+test_std,
        test_mean-test_std,
        alpha=0.15, color='green')
    
    plt.hlines(y=sigma, xmin=0, xmax=80, color='red', linewidth=2, linestyle='--')
    
    plt.title('Degree: %d' % d)
    plt.grid()
    plt.xlabel('Number of training samples')
    plt.ylabel('MSE')
    plt.legend(loc='upper right')
    plt.ylim([0, 3])
    plt.tight_layout()
    plt.savefig('./output/fig-learning-curve-%d.png' % d, dpi=300)
    plt.show()

We can see that in these regression tasks, a polynomial of any degree almost always overfits the training data when the model size is small, resulting in poor testing performance. This indicates that we should collect more data instead of sitting in front of the computer and play with the models. You may also try other models as different models has different sample complexity (i.e., number of samples required to successfully train a model).

Weight Decay

OK, we have verified the learning theory discussed in the lecture. Let's move on to the regularization techniques. Weight decay is a common regularization approach. The idea is to add a term in the cost function against complexity. In regression, this leads to two well-known models:

Ridge regression: $$\arg\min_{\boldsymbol{w},b}\Vert\boldsymbol{y}-(\boldsymbol{X}\boldsymbol{w}-b\boldsymbol{1})\Vert^{2}+\alpha\Vert\boldsymbol{w}\Vert^{2}$$

LASSO: $$\arg\min_{\boldsymbol{w},b}\Vert\boldsymbol{y}-(\boldsymbol{X}\boldsymbol{w}-b\boldsymbol{1})\Vert^{2}+\alpha\Vert\boldsymbol{w}\Vert_{1}$$

Let's see how they work using the Housing dataset:

import pandas as pd

df = pd.read_csv('https://archive.ics.uci.edu/ml/machine-learning-databases/'
                 'housing/housing.data',
                 header=None,
                 sep='\s+')

df.columns = ['CRIM', 'ZN', 'INDUS', 'CHAS', 
              'NOX', 'RM', 'AGE', 'DIS', 'RAD', 
              'TAX', 'PTRATIO', 'B', 'LSTAT', 'MEDV']
df.head()

	CRIM	ZN	INDUS	CHAS	NOX	RM	AGE	DIS	RAD	TAX	PTRATIO	B	LSTAT	MEDV
0	0.00632	18.0	2.31	0	0.538	6.575	65.2	4.0900	1	296.0	15.3	396.90	4.98	24.0
1	0.02731	0.0	7.07	0	0.469	6.421	78.9	4.9671	2	242.0	17.8	396.90	9.14	21.6
2	0.02729	0.0	7.07	0	0.469	7.185	61.1	4.9671	2	242.0	17.8	392.83	4.03	34.7
3	0.03237	0.0	2.18	0	0.458	6.998	45.8	6.0622	3	222.0	18.7	394.63	2.94	33.4
4	0.06905	0.0	2.18	0	0.458	7.147	54.2	6.0622	3	222.0	18.7	396.90	5.33	36.2

Remember that for weight decay to work properly, we need to ensure that all our features are on comparable scales:

from sklearn.preprocessing import StandardScaler

X = df.iloc[:, :-1].values
y = df['MEDV'].values

sc_x = StandardScaler()
X_std = sc_x.fit_transform(X)

Ridge Regression

We know that an unregularized polynomial regressor with degree $P=3$ will overfit the training data and has bad generalizability. Let's regularize its $L^2$-norm to see if we can get a better testing error:

from sklearn.linear_model import Ridge
from sklearn.metrics import mean_squared_error

poly = PolynomialFeatures(degree=3)
X_poly = poly.fit_transform(X_std)
X_train, X_test, y_train, y_test = train_test_split(
    X_poly, y, test_size=0.3, random_state=0)

for a in [0, 1, 10, 100, 1000]:
    lr_rg = Ridge(alpha=a)
    lr_rg.fit(X_train, y_train)

    y_train_pred = lr_rg.predict(X_train)
    y_test_pred = lr_rg.predict(X_test)

    print('\n[Alpha = %d]' % a )
    print('MSE train: %.2f, test: %.2f' % (
                    mean_squared_error(y_train, y_train_pred),
                    mean_squared_error(y_test, y_test_pred)))  

[Alpha = 0]
MSE train: 0.00, test: 19958.68

[Alpha = 1]
MSE train: 0.73, test: 23.05

[Alpha = 10]
MSE train: 1.66, test: 16.83

[Alpha = 100]
MSE train: 3.60, test: 15.16

[Alpha = 1000]
MSE train: 8.81, test: 19.22

We can see that a small value $\alpha$ drastically reduces the testing error. In addition, $\alpha = 100$ seems to be a good decay strength. As we can see, it's not a good idea to increase $\alpha$ forever, since it will over-shrink the coefficients of $\boldsymbol{w}$ and result in underfit.

Let's see the rate of weight decay as $\alpha$ grows:

X_train, X_test, y_train, y_test = train_test_split(
    X_std, y, test_size=0.3, random_state=0)

max_alpha = 1000
coef_ = np.zeros((max_alpha, 13))

for a in range(1, max_alpha):
    lr_rg = Ridge(alpha=a)
    lr_rg.fit(X_train, y_train)

    y_train_pred = lr_rg.predict(X_train)
    y_test_pred = lr_rg.predict(X_test)
    coef_[a,:] = lr_rg.coef_.reshape(1,-1)
    
plt.hlines(y=0, xmin=0, xmax=max_alpha, color='red', linewidth = 2, linestyle = '--')

for i in range(13):
    plt.plot(range(max_alpha),coef_[:,i])
    
plt.ylabel('Coefficients')
plt.xlabel('Alpha')
plt.tight_layout()
plt.savefig('./output/fig-ridge-decay.png', dpi=300)
plt.show()

LASSO

An alternative weight decay approach that can lead to sparse $\boldsymbol{w}$ is the LASSO. Depending on the value of $\alpha$, certain weights can become zero much faster than others, which makes the LASSO also useful as a supervised feature selection technique.

NOTE: since $L^1$-norm has non differentiable points, the solver (optimization method) is different from the one used in the Ridge regression. It will take much more time to train model weights.

from sklearn.linear_model import Lasso
from sklearn.metrics import mean_squared_error

X_train, X_test, y_train, y_test = train_test_split(
    X_poly, y, test_size=0.3, random_state=0)

for a in [0, 0.001, 0.01, 0.1, 1, 10]:
    lr_rg = Lasso(alpha=a)
    lr_rg.fit(X_train, y_train)

    y_train_pred = lr_rg.predict(X_train)
    y_test_pred = lr_rg.predict(X_test)

    print('\n[Alpha = %.4f]' % a )
    print('MSE train: %.2f, test: %.2f' % (
                    mean_squared_error(y_train, y_train_pred),
                    mean_squared_error(y_test, y_test_pred)))  

[Alpha = 0.0000]
MSE train: 0.55, test: 61.02

[Alpha = 0.0010]
MSE train: 0.64, test: 29.11

[Alpha = 0.0100]
MSE train: 1.52, test: 19.51

[Alpha = 0.1000]
MSE train: 4.34, test: 15.52

[Alpha = 1.0000]
MSE train: 14.33, test: 22.42

[Alpha = 10.0000]
MSE train: 55.79, test: 53.42

X_train, X_test, y_train, y_test = train_test_split(
    X_std, y, test_size=0.3, random_state=0)

max_alpha = 10
coef_ = np.zeros((max_alpha,13))

for a in range(10):
    lr_rg = Lasso(alpha=a+0.1)
    lr_rg.fit(X_train, y_train)

    y_train_pred = lr_rg.predict(X_train)
    y_test_pred = lr_rg.predict(X_test)
    coef_[a,:] = lr_rg.coef_.reshape(1,-1)
    
plt.hlines(y=0, xmin=0, xmax=max_alpha, color='red', linewidth = 2, linestyle = '--')

for i in range(13):
    plt.plot(range(max_alpha),coef_[:,i])
    
plt.ylabel('Coefficients')
plt.xlabel('Alpha')
plt.tight_layout()
plt.savefig('./output/fig-ridge-decay.png', dpi=300)
plt.show()

The result shows that as the $\alpha$ increases, the coefficients shrink faster and become exactly zero when $\alpha=8$.

LASSO for Feature Selection

Since we can choose a suitable regularization strength $\alpha$ to make only part of coefficients become exactly zeros, LASSO can also be treated as a feature selection technique.

var_num = X_train.shape[1]

lr_lasso = Lasso(alpha = 1)
lr_lasso.fit(X_train, y_train)
lr_ridge = Ridge(alpha = 1)
lr_ridge.fit(X_train, y_train)

plt.scatter(range(var_num),lr_lasso.coef_, label = 'LASSO', color = 'blue')
plt.scatter(range(var_num),lr_ridge.coef_, label = 'Ridge', color = 'green')
plt.hlines(y=0, xmin=0, xmax=var_num-1, color='red', linestyle ='--')
plt.xlim(0,12)
plt.legend(loc = 'upper right')
plt.xlabel('Coefficients index')
plt.ylabel('Coefficients')
plt.tight_layout()
plt.show()

epsilon = 1e-4
idxs = np.where(abs(lr_lasso.coef_) > epsilon)
print('Selected attributes: {}'.format(df.columns.values[idxs]))

Selected attributes: ['RM' 'TAX' 'PTRATIO' 'LSTAT']

We can plot the pairwise distributions to see the correlation between the selected attributes and MEDV:

import seaborn as sns

print('All attributes:')
sns.set(style='whitegrid', context='notebook')
sns.pairplot(df, x_vars=df.columns[:-1], y_vars=['MEDV'], size=2.5)
plt.tight_layout()
plt.show()

print('Selected attributes:')
sns.set(style='whitegrid', context='notebook')
sns.pairplot(df,x_vars=df.columns[idxs], y_vars=['MEDV'], size=2.5)
plt.tight_layout()
plt.show()
sns.reset_orig()

All attributes:

Selected attributes:

As we can see, LASSO extracted attributes that have more significant correlations with MEDV.

Ignoring Outliers using RANSAC

Linear regression models can be heavily impacted by the presence of outliers. In certain situations, a very small subset of our data can have a big effect on the estimated model coefficients, as shown below:

There are many statistical tests that can be used to detect outliers, which are beyond the scope of our course. However, removing outliers usually requires our human judgment as well as domain knowledge.

Next, we present the RANdom SAmple Consensus (RANSAC) algorithm for regression that can completely ignore the outliers and making them irrelevant to final predictions. RANSAC fits a regression model only to a subset of the data, the so-called inliers. RANSAC is an iterative algorithm, which can be summarized as follows:

1. Select a random number of samples to be inliers and fit the model.
2. Test all other data points against the fitted model and add those points that fall within a user-given tolerance to the inliers.
3. Refit the model using all inliers.
4. Estimate the error of the fitted model versus the inliers.
5. Terminate the algorithm if the performance meets a certain user-defined threshold or if a fixed number of iterations has been reached; go back to step 1 otherwise.

from sklearn.linear_model import RANSACRegressor

X = df['RM'].values[:, np.newaxis]
y = df['MEDV'].values

ransac = RANSACRegressor(LinearRegression(), 
            max_trials=100, 
            min_samples=50, 
            residual_threshold=16.0, 
            random_state=0)
ransac.fit(X, y)

inlier_mask = ransac.inlier_mask_
outlier_mask = np.logical_not(inlier_mask)
line_X = np.arange(3, 10, 1)
line_y_ransac = ransac.predict(line_X[:, np.newaxis])
plt.scatter(X[inlier_mask], y[inlier_mask], 
            c='blue', marker='o', label='Inliers')
plt.scatter(X[outlier_mask], y[outlier_mask],
            c='lightgreen', marker='s', label='Outliers')
plt.plot(line_X, line_y_ransac, color='red')
plt.xlabel('Average number of rooms [RM]')
plt.ylabel('Price in $1000\'s [MEDV]')
plt.legend(loc='upper left')
plt.tight_layout()
plt.show()
print('\n[RANSAC]')
print('Slope (w_1): {:.2f}    Intercept (w_0): {:.2f}'.format(ransac.estimator_.coef_[0],ransac.estimator_.intercept_))


slr = LinearRegression()
slr.fit(X, y)
print('\n[Ordinary least square]')
y_pred = slr.predict(X)
print('Slope (w_1): {:.2f}    Intercept (w_0): {:.2f}'.format(slr.coef_[0],slr.intercept_))

[RANSAC]
Slope (w_1): 10.22    Intercept (w_0): -41.93

[Ordinary least square]
Slope (w_1): 9.10    Intercept (w_0): -34.67

We set the maximum number of iterations of the RANSACRegressor to 100, and via the min_samples parameter we set the minimum number of the randomly chosen samples to be at least 50. Using the residual_metric parameter, we provided a callable lambda function that simply calculates the absolute vertical distances between the fitted line and the sample points. By setting the residual_threshold parameter to 5.0, we only allowed samples to be included in the inlier set if their vertical distance to the fitted line is within 5 distance units, which works well on this particular dataset. By default, scikit-learn uses the the Median Absolute Deviation (MAD) estimate of the target values $y$ to select the inlier threshold. However, the choice of an appropriate value for the inlier threshold is problem-specific, which is one disadvantage of RANSAC. Many different approaches have been developed over the recent years to select a better inlier threshold automatically.

Validation

Hyperparameters, i.e. constants in the model, may have impact on the model complexity and generalization performance. Validation is another useful regularization technique helping us decide the proper value of hyperparameters. The idea is to split your data into the training, validation, and testing sets and then select the best value based on the Occam's razor for validation performance. So, you don't peep testing set and report optimistic testing performance.

Let's follow the structural risk minimization framework to pick a right degree $P$ in polynomial regression:

from sklearn.linear_model import Ridge
from sklearn.preprocessing import StandardScaler

X = df.iloc[:, :-1].values
y = df['MEDV'].values

sc_x = StandardScaler()
X_std = sc_x.fit_transform(X)

for d in range(1, 7):
    poly = PolynomialFeatures(degree=d)
    X_poly = poly.fit_transform(X_std)

    X_train, X_test, y_train, y_test = train_test_split(
        X_poly, y, test_size=0.3, random_state=0)
    
    X_train, X_valid, y_train, y_valid = train_test_split(
        X_train, y_train, test_size=0.3, random_state=0)
    
    rg = Ridge(alpha=100)
    rg.fit(X_train, y_train)
    
    y_train_pred = rg.predict(X_train)
    y_valid_pred = rg.predict(X_valid)
    y_test_pred = rg.predict(X_test)
    
    print('\n[Degree = %d]' % d)
    print('MSE train: %.2f, valid: %.2f, test: %.2f' % (
                    mean_squared_error(y_train, y_train_pred),
                    mean_squared_error(y_valid, y_valid_pred),
                    mean_squared_error(y_test, y_test_pred))) 

[Degree = 1]
MSE train: 25.00, valid: 21.43, test: 32.09

[Degree = 2]
MSE train: 9.68, valid: 14.24, test: 20.24

[Degree = 3]
MSE train: 3.38, valid: 17.74, test: 18.63

[Degree = 4]
MSE train: 1.72, valid: 16.67, test: 30.98

[Degree = 5]
MSE train: 0.97, valid: 59.73, test: 57.02

[Degree = 6]
MSE train: 0.60, valid: 1444.08, test: 33189.41

We pick $P=2$ because it gives a low enough validation error at the simplest complexity. Then, we report the testing error over the test set. In general, you may not be able to pick the $P$ that gives the lowest testing performance. In this case you get the 20.24% testing error instead of 18.63%. But that's the right way to do error reporting.

Assignment

In this assignment, we would like to predict the success of shots made by basketball players in the NBA.

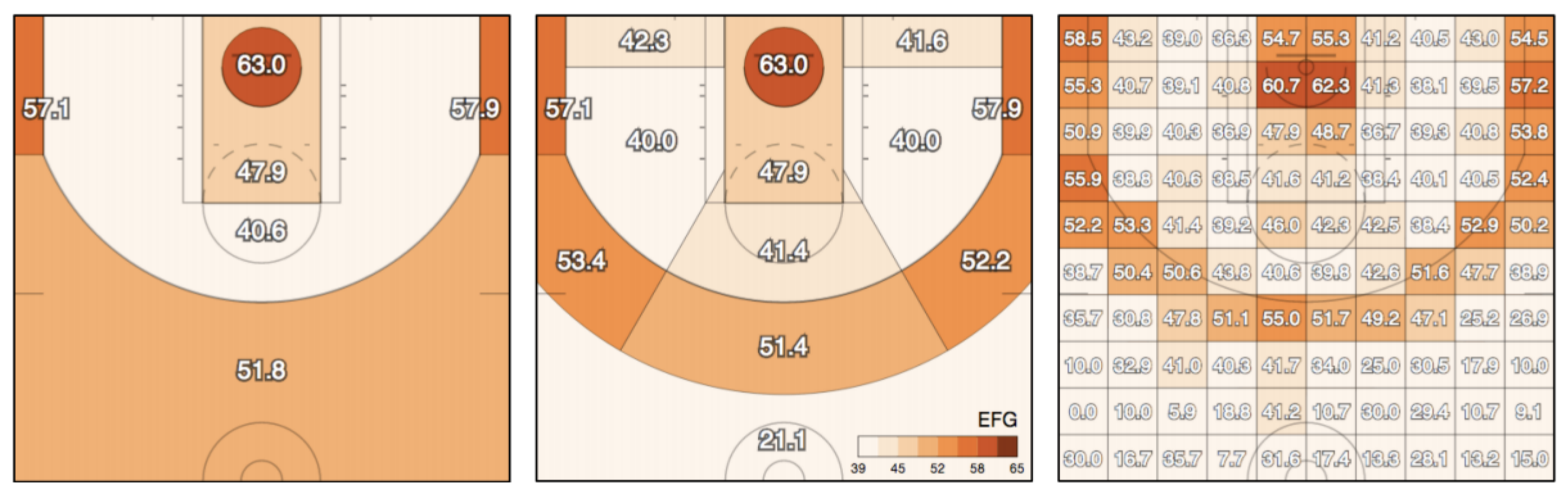
Please download the dataset first. You might need to try with various models to achieve better performance.

Requirements

• Submit to eeclass with your code file Lab05_{student_id}.ipynb (e.g. Lab05_109069999.ipynb) and prediction file Lab05_{student_id}_y_pred.csv. The notebook should contain the following parts:
  1. Use all features to train any linear model in scikit-learn and try different hyperparameters (ex. different degree, complexity). Show their performances.
  2. Select 1 setting (model + hyperparameters) and plot the error curve to show that the setting you selected isn't over-fit.
  3. Use any method to choose the best 3 features that can best aid the model's prediction. Explain how you find it.
     • Note: the combination of features doesn't count as 1 feature, e.g. $x_1, x_2,$ and $x_1^2+x_2$ count as only two features.
  4. Train the model selected in 2. with the only 3 features selected in 3., and present the training error.
  5. Export the predictions of the model trained in 4. for X_test (follow the format of y_train.csv).
  6. A brief report of what you have done in this assignment.
• Prediction performance will have minimal impact on the assignment grade, so there's no need to be overly concerned about it.
• Deadline: 2024-10-2 (Wed) 23:59.

Hints

1. You can preprocess the data to help your training.
2. Since you don't have y_test this time, you may need to split a validation set for checking your performance.
3. It is possible to use a regression model as a classifier, for example RidgeClassifier.

References

1. Stanford CS229 Machine Learning
2. NBA shot logs

import pandas as pd
import numpy as np

# download the dataset
import urllib.request

urllib.request.urlretrieve("https://nthu-datalab.github.io/ml/labs/05_Regularization/data/X_train.csv", "./data/X_train.csv")
urllib.request.urlretrieve("https://nthu-datalab.github.io/ml/labs/05_Regularization/data/y_train.csv", "./data/y_train.csv")
urllib.request.urlretrieve("https://nthu-datalab.github.io/ml/labs/05_Regularization/data/X_test.csv", "./data/X_test.csv")

X_train = pd.read_csv('./data/X_train.csv')
y_train = pd.read_csv('./data/y_train.csv')
X_test = pd.read_csv('./data/X_test.csv')

print(X_train.shape)
print(X_train.columns)
print(y_train.columns)

(85751, 8)
Index(['PERIOD', 'GAME_CLOCK', 'SHOT_CLOCK', 'DRIBBLES', 'TOUCH_TIME',
       'SHOT_DIST', 'PTS_TYPE', 'CLOSE_DEF_DIST'],
      dtype='object')
Index(['FGM'], dtype='object')

X_train.head()

	PERIOD	GAME_CLOCK	SHOT_CLOCK	DRIBBLES	TOUCH_TIME	SHOT_DIST	PTS_TYPE	CLOSE_DEF_DIST
0	1	358	2.4	0	3.2	20.6	2	4.5
1	1	585	8.3	0	1.2	3.0	2	0.5
2	1	540	19.9	0	0.6	3.5	2	3.2
3	1	392	9.0	0	0.9	21.1	2	4.9
4	3	401	22.7	0	0.7	4.1	2	2.9